Definition Of Harmonic Function In Complex Analysis
Harmonic functions appear regularly and play a fundamental role in math physics andengineering. U R 2 R 2 satisfying u v 0.

Pic 1 Fourier Series We Have Learnt A Bit About The Needed Basics Before Beginning The Fourier Series And The Eule Mathematics Differential Equations Cymatics
In fact to a large extent complex analysis is the study of analytic functions.

Definition of harmonic function in complex analysis. There are two different types of singular points. When this happens we say that the function f is. The real and imaginary parts of an analytic function are harmonic conjugate functions ie solutions to Laplace equation and satisfy the Cauchy Riemann equations 2 3.
U x2 y2- 2z2 Ω Ɍ3u 1r Ω Ɍ3- 0 0 0 where r x2 y2z2. Save Word Definition of harmonic analysis. Let fz uxyivxy f z u x y i v x y be a analytic function then f z is said to be harmonic and it satisfies to the Laplace equation ie.
Complex analysis traditionally known as the theory of functions of a complex variable is the branch of mathematical analysis that investigates functions of complex numbers. In the next topic we will look at someapplications to. Other sets by this creator.
The definition of a Harmonic function Harmonic conjugate function and how Analytic functions and Harmonic functions are related through some theorems. Definition of Analytic Function Harmonic Function. The expression of a periodic function as a sum of sines and cosines and specifically by a Fourier series Examples of harmonic analysis in a Sentence.
Throughout this booknwill denote a fixed positive integer greater than 1 and Ω will denote an open nonempty subset of RnA twice continuously differentiable complex-valued functionudefined on Ω isharmonicon Ω if u0 whereD1 2Dn 2andDj. If f x i y u x y i v x y is analytic on a region u and v are harmonic and we also have u v 0. To go the other way given harmonic u v.
The key connection to 1804 is that both the real andimaginary parts of analytic functions are harmonic. Analytic and Harmonic Functions 31 Differentiable Functions Letbe a complex function that is defined at all points in some neighborhood of zo-The derivative of fat zo is written fzo and is defined by the equation 1 zo lim zz Z Zo provided that the limit exists. 3 Singularities of Analytic Functions Points at which a function fz is not analytic are called singular points or singularities of fz.
Start studying Complex Analysis. More to this considering Ω an open set in Ɍ3 the real part of the analytic function is always harmonic. Construction of Analytic Function.
It is useful in many branches of mathematics including algebraic geometry number theory analytic combinatorics applied mathematics. Those 2 properties of complex integrals. We will see that this is a simpleconsequence of the Cauchy-Riemann equations.
After a brief review of complex numbers as points in the complex. A function u is called harmonic if Δ u 0. As well as in physics including the branches of hydrodynamics thermodynamics and particularly quantum mechanics.
HARMONIC FUNCTIONS FROM A COMPLEX ANALYSIS VIEWPOINT SHELDON AXLER Department of Mathematics Michigan State University East Lansing MI 48824 Recall that a real valued function u defined on an open set in the complex plane is called harmonic if the partial second derivatives of u exist and are continuous and uxx uyy is identically zero. Harmonic u and v satisfying this condition are called harmonic conjugates. 0 u x x y dy.
This video Covers following topics of Unit-I Engg Mathematics-III1. Now v x x y. Harmonic function mathematical functionof two variables having the property that its value at any point is equal to the average of its values along any circle around that point provided the function is defined within the circle.
In this topic well learn the denition some key properties and their tightconnection to complex analysis. By extension use of complex analysis. Expz sinz cosz formulae.
Thats all there is to it. Instrumental Methods Chapter. To the case of complex functions of a complex variable.
Since u is harmonic function u have continuous pa rtial derivative. Harmonic functions also occur as the potential functions for two-dimensional gravitational electrostatic and electromagnetic fields in regions of space which are respectively free. Harmonic Functions The following are basic examples of harmonic functions.
Upto 2nd order and u xx u yy 0 on r 0. Eust Lunsirzg M I 48824 Recall that a real valued function u defined on an open set in the complex plane is called harmonic if the partial second derivatives of u exist and are continuous and u U. V x y Z x.
Learn vocabulary terms and more with flashcards games and other study tools. In so doing we will come across analytic functions which form the centerpiece of this part of the course. Harmonic functions for us live on open subsets of real Euclidean spaces.
MARMONPC FUNCTIONS FROM A COMPLEX ANALYSIS VIEWPOINT SHELDON AXLER Depurtnentof Muthemurics Michigan Stute Uniuersitj. 0 u y x 0 dx Z y.

Relations Between Polar Coordinates Spherical Harmonics Cartesian Coordinates Anatomy And Flight Mecha Cartesian Coordinates Spherical Harmonics Coordinates

Representation Theory And Noncommutative Harmonic Analysis Ii Pdf Analysis Theories Mathematics

Harmonic Functions A Quick Proof Complex Analysis 4 Youtube
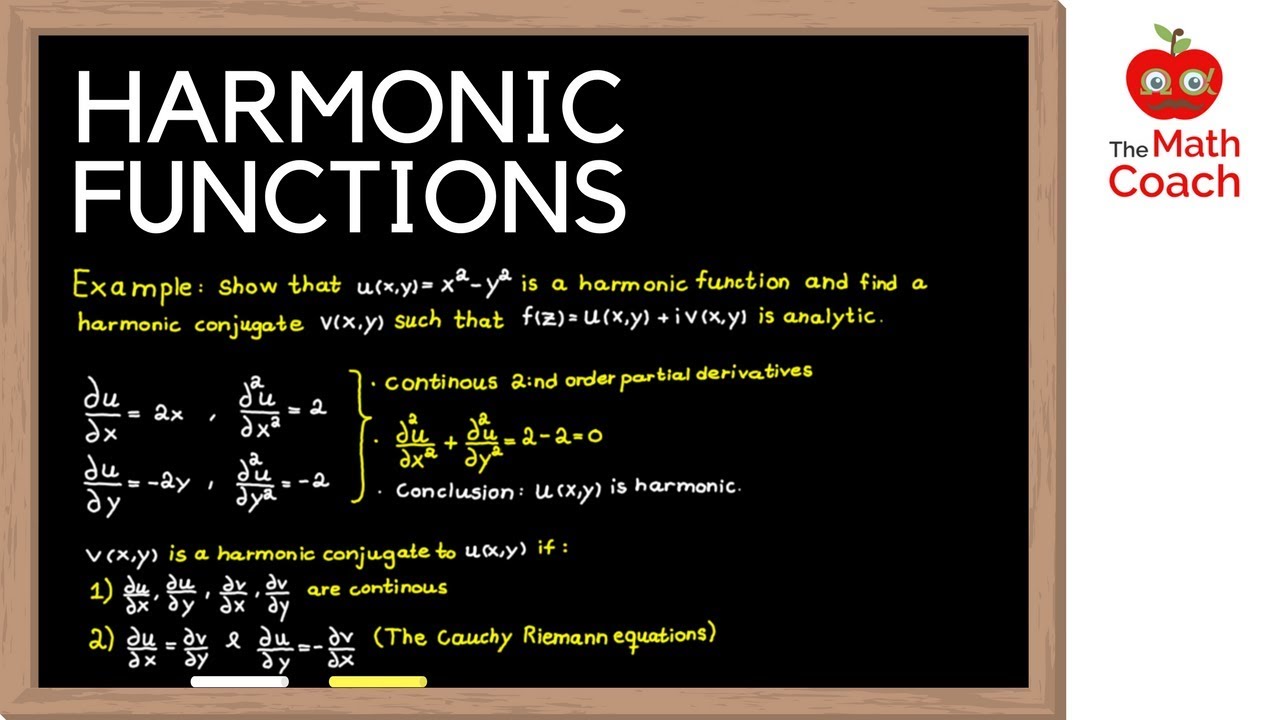
Harmonic Functions Harmonic Conjugate Complex Analysis 3 Youtube

About The Existence Of Harmonic Conjugate Mathematics Stack Exchange

Fourier Analysis Spherical Harmonics Google Search Spherical Harmonics Euclidean Space Hilbert

Harmonic Functions Harmonic Conjugate Complex Analysis 3 Youtube

Sum Of Harmonic Numbers Induction Proof Math Videos Maths Exam Mathematical Induction

7 Harmonic Function Problem 1 Complete Concept Youtube
Https Math Mit Edu Jorloff 18 04 Notes Topic5 Pdf
Https Math Mit Edu Jorloff 18 04 Notes Topic5 Pdf
Harmonic Function Mathematics Britannica
Https Math Mit Edu Jorloff 18 04 Notes Topic5 Pdf

The Symmetry Of Standard Normal Distribution In Calculus Terms Calculus College Math Math
Https Math Mit Edu Jorloff 18 04 Notes Topic5 Pdf
Https Math Mit Edu Jorloff 18 04 Notes Topic5 Pdf

Complex Analysis 04 Harmonic Functions Youtube

Every Real Valued Analytic Function In A Domain Is Constant Proof Math Videos Maths Exam Complex Analysis

Post a Comment for "Definition Of Harmonic Function In Complex Analysis"